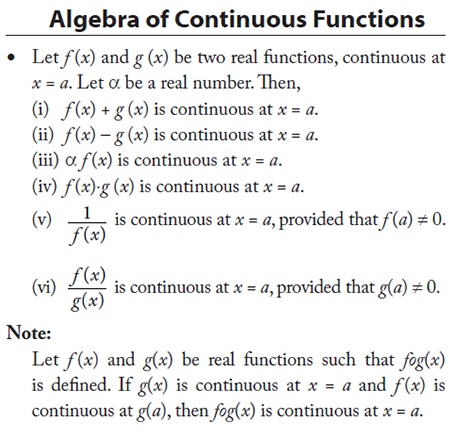
Q 3185012867
 Discuss the continuity of the function f defined by `f (x) = 1/x , x ≠ 0` .
Discuss the continuity of the function f defined by `f (x) = 1/x , x ≠ 0` .
Class 12 Chapter 5 Example 9

Class 12 Chapter 5 Example 9

Solution:
Fix any non zero real number c, we have
` lim_(x->c) f(x) = lim_(x->c) 1/x = 1/c`
Also, since for `c ≠ 0, f (c) = 1/c`, we have ` lim_(x->c) f(x) = f(c)` and hence, f is continuous
at every point in the domain of f. Thus f is a continuous function.
We take this opportunity to explain the concept of infinity. This we do by analysing
the function `f (x) =1/x` near x = 0. To carry out this analysis we follow the usual trick of
finding the value of the function at real numbers close to 0. Essentially we are trying to
find the right hand limit of f at 0. We tabulate this in the following (Table 5.1).
We observe that as x gets closer to 0 from the right, the value of f (x) shoots up
higher. This may be rephrased as: the value of f (x) may be made larger than any given
number by choosing a positive real number very close to 0. In symbols, we write
`lim_(x->0^+) f(x) = + oo`
(to be read as: the right hand limit of f (x) at 0 is plus infinity). We wish to emphasise
that + ∞ is NOT a real number and hence the right hand limit of f at 0 does not exist (as
a real number).
Similarly, the left hand limit of f at 0 may be found. The following table is self
explanatory.
From the Table 5.2, we deduce that the
value of f (x) may be made smaller than any
given number by choosing a negative real
number very close to 0. In symbols,
we write
`lim_(x-> 0^-) f(x) = - oo`
(to be read as: the left hand limit of f (x) at 0 is
minus infinity). Again, we wish to emphasise
that – ∞ is NOT a real number and hence the
left hand limit of f at 0 does not exist (as a real
number). The graph of the reciprocal function
given in Fig 5.3 is a geometric representation
of the above mentioned facts.
Fix any non zero real number c, we have
` lim_(x->c) f(x) = lim_(x->c) 1/x = 1/c`
Also, since for `c ≠ 0, f (c) = 1/c`, we have ` lim_(x->c) f(x) = f(c)` and hence, f is continuous
at every point in the domain of f. Thus f is a continuous function.
We take this opportunity to explain the concept of infinity. This we do by analysing
the function `f (x) =1/x` near x = 0. To carry out this analysis we follow the usual trick of
finding the value of the function at real numbers close to 0. Essentially we are trying to
find the right hand limit of f at 0. We tabulate this in the following (Table 5.1).
We observe that as x gets closer to 0 from the right, the value of f (x) shoots up
higher. This may be rephrased as: the value of f (x) may be made larger than any given
number by choosing a positive real number very close to 0. In symbols, we write
`lim_(x->0^+) f(x) = + oo`
(to be read as: the right hand limit of f (x) at 0 is plus infinity). We wish to emphasise
that + ∞ is NOT a real number and hence the right hand limit of f at 0 does not exist (as
a real number).
Similarly, the left hand limit of f at 0 may be found. The following table is self
explanatory.
From the Table 5.2, we deduce that the
value of f (x) may be made smaller than any
given number by choosing a negative real
number very close to 0. In symbols,
we write
`lim_(x-> 0^-) f(x) = - oo`
(to be read as: the left hand limit of f (x) at 0 is
minus infinity). Again, we wish to emphasise
that – ∞ is NOT a real number and hence the
left hand limit of f at 0 does not exist (as a real
number). The graph of the reciprocal function
given in Fig 5.3 is a geometric representation
of the above mentioned facts.